Integration
Integration is the counterpart, or the reverse process of differentiation,both of which are the techniques of calculus that were developed by Newton and Leibnitz. While differentiation deals with measurement of the rate of change in a dependent variable with reference to a very small change (approaching almost zero) in its relate independent, variable, integration deals with the calculation of the total amount of changes in the given range of the values. In calculation of the total amount of changes in the given range of the values. In other words, with the technique of integration, we find the functional relationship between any two, or more variables from the rate of change existing between them, whereas, with the technique of differentiation, we find the rate of change between some variables from a given functional relationship existing between them.
Hence, integration is just the opposite of the differentiation. Thus, when f(x) = x and differentiation, or d/dx(x) = 1, then its integration, or f 1 dx = x + C.
Where c represents a constant whose d/dx = 0
Similarly,
When (f(x) = x3, and d/dx (x3) = 3x2, then its integration, or f 3x2dx = x3 + c
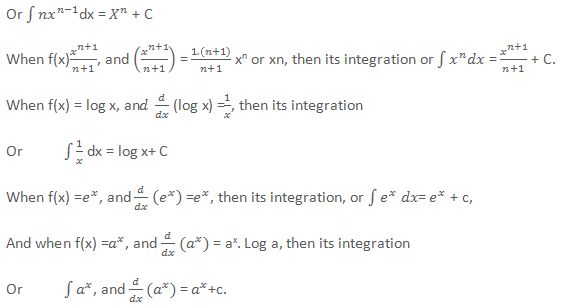
When f(x) = xn, and d/dx (xn) = nxn-1, then its integration
From all the above examples, it must be clear that integration of the derivation, or differentiation of a function itself. Hence, integration of a derivative is otherwise called the anti-derivative, primitive, or integral of a function.