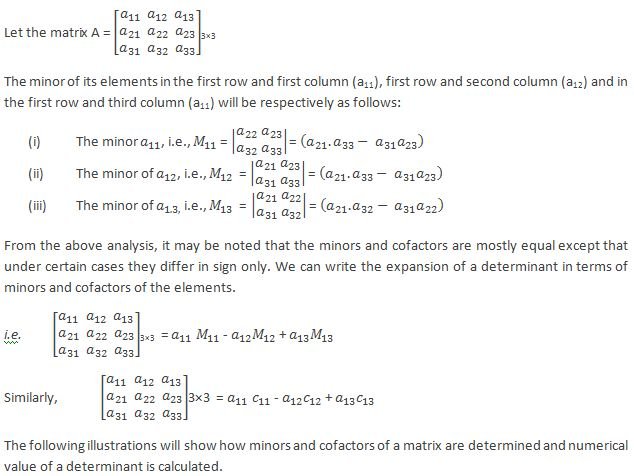
Co Factors
By the co factor of an element of a matrix we mean the product of (-1)i+j and the minor of the concerned element (Mij)
Where Cij = the co factor of the element in the ith row and jth column of the matrix.
(-1)i+j =the factor determining the algebraic sign depending upon the number of row (i) and number of column (j) in which the element occurs in the matrix.
Mij = the minor of the element in the ith row and jth column of the matrix.
Thus, C11 = (-1)1+1, m11; c12 = (-1)1+2. M12, and c13 = (-1)1+3. M13
Minor
By the minor of an element of a matrix we mean the sub square-matrix of the given matrix along which the particular element (eij) does not exist. It is obtained by deleting the row and the column on which the particular element (eij) lies. It is representing by Mij, which denotes the minor of an element in the ith row and jth column of the matrix. Its value is obtained by deducting the product of its non-leading diagonal elements from the product of its leading diagonal elements.
Examples: