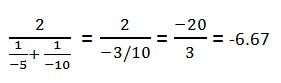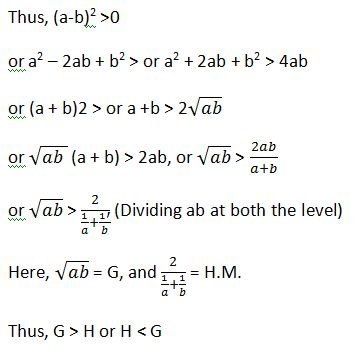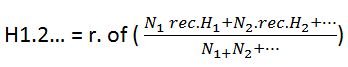Algebraic Property of Harmonic Mean

Harmonic mean as a mathematical average as a lot of algebraic properties. Some important ones of which are enumerated as under

1. If any two of the three factors viz. H,N and  ∑ r (X) are given , the value of the third unknown factor can be found out.

 Thus, H = N/∑ r (x)

=N = H. ∑ r (x)

= ∑ r (x) = N/H

2. Harmonic mean can be computed from a series with any number of negative values.

Thus, the harmonic mean of the values: -5 and -10 will be

3. Harmonic mean cannot be computed from a series if any its values is zero.This is because, the reciprocal of 0 does not exist i.e.  = infinity.

4. For any series in which all the values are not equal nor any equal nor any value is zero, the value of the harmonic mean is less than the geometric mean and arithmetic mean.

Proof :

Let ‘a’ be the largest value and ‘b’ be the smallest value of a series.

5. The harmonic mean of a number of series can be computed in a computed manner, if the harmonic mean, and the number of items of each of such series are given, Thus