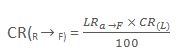Link Relative Method

This method of finding the seasonal indices in the form of the chain relatives was developed by Prof. Karl Pearson, and hence, this method is also known as the Pearson method of seasonal variation. Under this method, the seasonal indices are found out with the following steps in turn:

Steps

(i) Find the link relatives of all the seasonal data by the formula,

LR1 = (m1/m0) x 100

Where, LR1 = line relative of the current season

m1 = data of the current season

And  m0 = data of the immediately preceding season (the link relative for the first and foremost season being nil.)

(ii) Arrange all the link relatives thus obtained season wise and find the seasonal average of such link relatives either by Mean or by Median.

(iii) Convert each of the averages of the link relatives into chain relatives by the formula,

Where,     CR1 = chain relative of the current season,

L.R._(a)1 = average of the link relative of the current season:

And CR0 = chain relative of the immediately preceding season

 (the initial chain relative of the foremost season being 100.)

(iv) Find the revised chain relative of the foremost season on the basis of the chain relative of the last season by the formula,

CR(_{R → F)}  = the revived chain relative of the fire most season.

LR(a _{→ F) =} the average link relative of the foremost season, and

CR(L) = the chain relative of the last season.

(v) Determine the constants correcting the chain relatives by d =

Where, d = the difference between the two chain relatives of the foremost season that is used as the correction factor;

CR(_{R → F) =} the revised chain relative of the foremost season;

CR(i_{→ F) =} the initial chain relative of the foremost season;

n = the number of season in a year (i.e. 12 months, or 4 quarters and so on)

(vi)  Deduction of the correction factor d, after being multiplied by 1,2, 3 (and so an) respectively from the chain relatives of the 2nd, 3rd, 4th and so on seasons, and thereby find the preliminary indices of the seasonal variations.

(vii)  Express the preliminary indices as the percentage of their average to get the required seasonal indices. Hence, the seasonal variation is nothing but the corrected chain relatives expressed as percentage of their averages.