Measures of Skewness

Like the measures of dispersion, Skewness can be computed in two ways viz. absolute and relative. The relative skewness is otherwise called coefficient of skewness. For a comparative study of more than one distribution, coefficient of skewness is found out.

However, there are different measures of skewness propounded by different authors on the basis of the different tests if skewness as under:

General measures of Skewness

(a)    Absolute skewness.

This is obtained by finding the difference between any two measures of dispersion viz: Mean, Median and More. Thus, Skewness or

Sk =

X –M

or  X – Z

or M-Z

Any positive value obtained by any of the above formulae is marked as the extent of the positive skewness. Any negative value obtained by any of the above formulae is marked as the extent of the negative skewness of the distribution. If the result produced is zero, it signifies the absence of skewness in the distribution.

(a)    Co-efficient of skewness

 This is obtained by dividing the Skewness by any measure of dispersion.

Thus,

2. Karl Pearson’s Skewness

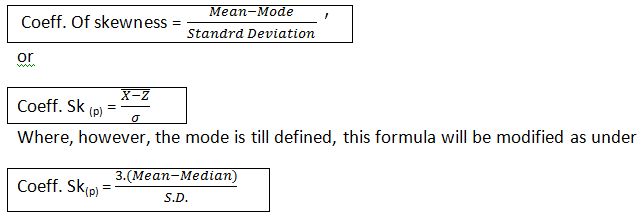
Prof. Karl pearson says that to study the skewness of a series, the difference between the Mean and Mode only should be found out. This is because, Mean is an average which is affected very much by the extreme values of a series and Mode is an average which is least affected by the extreme values of a series. Thus, according to him,

Sk_(p) = Mean –Mode

When, Mode is ill defined i.e. when it has different values, Prof. Pearson proposes to find out the skewness by the following formula:

Sk_(p) = 3 (Mean –Median)

This formula is based on the empirical relationship between Mean, Median and Mode which is as follows:

Absolute              Mode= 3 Median – 2 Mean

Thus,     Sk(p)      = X¯ – (3M- 2X¯)

                                =  X¯ – 3M +2X¯

                                = 3X¯ – 3M

                                = 3(X¯ -M)

For finding the coefficient of skewness, Prof. Pearson advocates that only standard diviation should be taken as the divisor of the absolute skewness. This is because, standard deviation is the only measure of dispersion which possesses many algebraic properties, and other measures of dispersion are not capable of algebraic treatments. Thus, his coefficient of skewness is given by

Limits of the results

Prof. Pearson claims that the results of his coefficient of skewness shall lie withing ± 3.

3. Bowley’s Skewness

According to Prof. A.L. Bowley, the presence, or absence of skewness will be determined on the basis of the distance of the quartiles from the Median. Thus, his skewness is given by

                SK(B) = (Q₃ – M) – (M –Q₁)

                Or     = Q₃ + Q₁ -2M

If the above equation results in zero, it will indicate the absence of skewness or symmetricity of the distribution. On the other hand, if the said equation results in some positive, or negative figure, the same will be marked as the extent of the positive, or negative skewness of the series respectively.

Further, the coefficient, the coefficient of skewness of Prof. Bowley is given by

4. Kelley’s Skewness

Prof. Kelley says that the above measure of skewness as propounded by Prof. Bowely ignores the first 25% of the data by measuring the skewness on the basis of the distance of the two extreme Quartiles form Median. To minimize the magnitude of such avoidance of the data, he advocates that the skewness should be measured on the basis of the distance of the two extreme Deciles, or Percentiles from the Median. Thus, according to him, the skewness is given by

SK (K) = (D₉ – M) – (M –D₁)

                                = D₉ + D₁ -2M

                                = (P₉₀ –M) –(M-P₁₀)

                                = P₉₀ + P₁₀ – 2M

Accordingly, the coefficient of skewness of Prof. Kelley is given by